
by:
Brandt Hacker
In this assignment we are asked to examine the following
scenario: Given two circles
and a point on one of the circles. Construct a circle tangent to the two
circles with one point of tangency being the designated point.
In order to tackle this question we must first understand what tangent
circles are. Two circles are said
to be tangent to one another if they intersect at a single point. If you would like to see a further
explanation of this concept as well as several illustrations of tangent
circles, click http://en.wikipedia.org/wiki/Tangent_circles.
The problem asks us to construct a circle tangent to two other
circles. We will attempt to do
this by first drawing our two circles.
Then, we will draw a line through center of circle A that passes through
the point C which we had drawn on the circumference of circle A. After constructing the line through
points A and C, then construct a circle with the same radius as circle B,
centered at C.
1. 2.
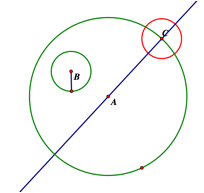

The two original circles are pictured in green. We will use the newly created red
circle to try and accomplish the original goal of creating a circle that is
tangent to both circle A and circle B.
After completing the constructions seen above, we will then mark the
point of intersection between circle C and line AC outside the circle, letŐs
call this point D. After creating
point D, we will then want to make a perpendicular bisector of the segment
running from B to C, the intersection o this perpendicular bisector and line AC
is the center of our tangent circle (pictured in black).
3. 4.


Below is an image of the tangent circle with the rest of the
construction hidden:

We will now use this construction to examine what happens to the center
of the tangent circle as we rotate point C around circle A. For GeometerŐs Sketchpad file that
allows you do this on your own, click HERE(A7tangentinside).
For the first scenario we will look at what happens as we rotate point
C around circle A when circle B is inside circle A. We will trace point E, the center of
the tangent circle, as we rotate point C around circle A. When traced we see that point E creates
an ellipse with points B and A, the centers of the two original circles, as the
foci of the ellipse.

Secondly, we will look at what happens as we rotate point C around
circle A when circle B intersects circle A. We will trace point E, the center of
the tangent circle, as we rotate point C around circle A. When traced we see that point E, once
again, creates an ellipse with points B and A, the centers of the two original
circles, as the foci of the ellipse.

Lastly, we will look at what happens as we rotate point C around circle
A when circle B is located outside circle A. We will trace point E, the center of
the tangent circle, as we rotate point C around circle A. When traced we see that this time,
point E creates a hyperbola with points A and B as the foci of the hyperbola.
